Edafología. Volumen 7-1. Abril 2000. pág 107-119.
A BASIC THERMODYNAMIC VOCABULARY
GEOCHEMISTRY IN THE ZONE OF SOIL FORMATION
APPLICATIONS OF THE PHASE RULE TO SOIL SYSTEMS
PROBLEMS WITH THE APPLICATION OF THE PHASE RULE TO SOILS
The Phase Rule was an incidental offshoot of the classic investigations in which J. Willard Gibbs laid out the foundations of chemical thermodynamics between 1875 and 1878. A reprint exists (Gibbs, 1961) together with two helpful commentaries (Donnan and Haas, 1936; Seeger, 1974). Connolly (1990) gives a thorough discussion of multivariable phase diagrams and includes a useful account of thermodynamic nomenclature. Regarding phase equilibria in aqueous systems under earth-surface conditions (Pankow, 1999) should be consulted.
Gibbs' Phase rule provides a theoretical basis for considering problems concerning the mineralogy of soils. In a deductive way, it serves as a check and a balance to the science of pedology, which like all the earth sciences is fundamentally inductive in nature. Since the Phase Rule has its derivation in classical thermodynamics it deals with systems at equilibrium. As soils are manifestly in a state of disequilibrium, application of the Phase Rule (or thermodynamics in general) needs some initial justification. First, the equilibrium state represents the state a system would achieve given the time and energy to get there. As such the equilibrium model lays fundamental constraints on any hypothesis of mineral genesis in soils. It indicates the direction of change, and places an end bracket on all states the system might pass through. In some cases, an equilibrium mineralogy may be approached closely, for example in microscopic systems ( Chesworth and Dejou, 1980) and as a result of long term weathering in the humid tropics. The model it provides is a rigorous one and although the natural, disequilibrium state can be expected to differ from the model, the differences are themselves instructive.
As a guide to what mineralogical equilibria are likely to be of interest in the present context, a brief review of the geochemistry of the zone of soil formation will be necessary. This follows a basic introduction to the terminology of thermodynamics and of phase equilibria.·
A BASIC THERMODYNAMIC VOCABULARY
The following terms are necessary in any discussion of phase equilibria. Morel (1983) provides a thorough examination of each term.
SYSTEM: An arbitrary part of the universe considered as an entity. There are three types: isolated systems have boundaries that allow neither mass nor energy transfer with the surroundings; closed systems exchange energy but not mass with the surroundings; open systems exchange both energy and mass with the surroundings. The word is also used in a purely chemical sense as in the phrase 'the system SiO 2 -A1 2 O 3 -H 2 O' where it means all possible thermodynamic systems made up these specific components.
SURROUNDINGS: that part of the universe lying outside the boundaries of the arbitrary system. The environment of the system.
PHASE: a physically homogeneous, and physically separable part of a system.
COMPONENTS: the smallest number of independently variable chemical entities needed to express the composition of a system, all phases within it, and all reactions that take place there.
STATE FUNCTION: properties or parameters that define the state of a system and are independent of the path by which the system reached its state. There are two types: intensive, which are independent of mass (e.g. pressure, temperature, chemical potential); and extensive, which are proportional to mass (e.g. volume, entropy, internal energy).
PATH FUNCTION: properties or parameters that depend upon the path a system follows to reach its current state (e.g. work, heat used in the process).
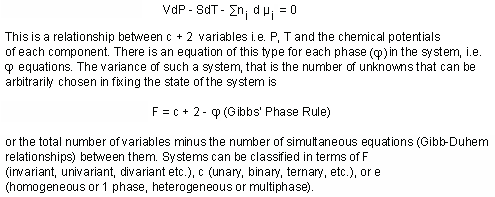
VARIANCE: the number of intensive parameters which must be arbitrarily fixed to define the system. Also called the number of degrees of freedom.
For a phase at equilibrium in a system, disregarding the presence of external fields (gravitational, electrical, magnetic), considering work of only PV type, and ignoring all surface and boundary effects, the Gibbs-Duhem equation holds:

Choice of components may represent some difficulty since, in the derivation of the Phase Rule, it is their number and not their nature that is significant. The first thing to realize is that a component need to have no independent existence as such. Indeed a component may be entirely fictive, for example, the component NaK -1 which exchanges K with Na in a mineral structure. The fact that oxide components are often used by earth scientists is a reflection of convenience and of history; mineral and rock analyses have usually been given in weight percentages of oxide constituents, since the old days of gravimetric analysis. The major point to stress in Gibbs' usage, is that the components chosen must be independently variable of each other and must represent the smallest possible number. Prigogine and Defay (1954) use the term differently in defining components as the chemical variables whose masses sum to the total mass of the system. When the number of reactions between the components in this sense, is subtracted form the number of components, the equivalent of Gibbs' independently variable components is obtained.
A further point of importance is the difference between inert and perfectly mobile components, terms introduced by Korzhinskii (1959) but inherent in Gibbs invention of the chemical potential determined inside the system of interest, whereas a perfectly mobile component has its chemical potential applied from outside, in analogous fashion to pressure. Zen (1963) offers a commentary on this distinction.
A phase diagram is a graphic representation of phase equilibria with a one to one geometrical correspondence with the phase rule. Geometrically, a dimensionless point is the equivalent of an invariant system ( c + 2 phases). A univariant system ( c + 1 phases) becomes a line or curve, a divariant system ( c phases) becomes an area, and a trivariant system ( c - 1 phase) a volume. Systems of greater variance can only be shown diagrammatically with one or more variables held constant. In a Cartesian depiction of two variables, an invariant point would have c + 2 univariant curves disposed about it, delimiting c + 2 divariant areas between the curves. The one component system CaCO 3 illustrates these points (figure 1.)
Of particular usefulness in the study of soil mineralogy are chemical potential diagrams or their equivalents which use activity or fugacity as variables (Garrels and Christ 1965, Lindsay 1979, Bowers et al, 1984). Figure 2 is a polybaric, polythermal diagram of the system A1 2 O 3 -S i O 2-H 2 O. Since the activity of Al 3+ and pH are related by equations such as A1(OH)3 (gibbsite) + 3H + = A1 3+ + 3H 2 O A1 2 -Si 2 O 5 (OH)4 (kaolinite) + 6H + = 2A1 3+ + 2H 4 SiO 4 + H 2 O in which A1 3+ and H + show a constant 1:3 stoichiometric correspondence, the solution saturated equilibria in this system, under isobaric, isothermal conditions, can be plotted in terms of the variables shown in figure 3.
GEOCHEMISTRY IN THE ZONE OF SOIL FORMATION
Geochemically, the soil producing system is one of mineral-water interaction. The mineral component can be looked upon as an intrinsic, and the water as an extrinsic or environmental factor. Intrinsic factors are those dictated by the parent material, and for most soils, the mineral substrate inherited from the parent material is either aluminosilicate or carbonate in nature with sulphides a notable feature when present. Figure 1 places the principal soil forming minerals into a stability sequence, and indicate some of the isomorphous replacements that are possible.
The agent by which the minerals are weathered and transformed, is the aqueous phase and it is through the aqueous phase that the chemical effects of climatic, biological, anthropic, watershed characteristics and other environmental factors principally make themselves felt. This aqueous environment at the earth's surface can conveniently be defined in terms of the parameters pe (or Eh) and pH. Within a pe-pH framework three major trends can be delineated (Fig.1, and Chesworth, 1992).
1. The acid trend, the common trend of weathering, is found in humid climates on materials with a generally unimpeded drainage. Movement of water in the system is predominantly downwards, hydrolysis is the principle chemical reaction taking place, and leaching, with or without movement of an entrained clay fraction (illuviation), is the main process modifying the bulk chemistry of the soil. A titration of the aqueous phase, acidified by atmospheric gases and acids of organic origin, against aluminosilicates and carbonates of the common rocks, leads to the progressive protonization of the terrestrial surface, the displacement of cations and silica from mineral structures, the production of secondary phases and groundwaters of predominantly bicarbonate type. Three types of pedogenesis are produced in this trend: polzolisation, principally in temperate zones, with a significant mobilization of A1 and Fe as organo-metallic complexes; ferrallitization wherein A1 and Fe accumulate relative to Si in surface horizons; and andosolization where the presence of glassy parent materials tends to produce large amounts of amorphous alumino-silicate phases. All three pedogeneses have a tendency to move towards compositions and mineral assemblages in the relatively simple 4-component system SiO 2 -A1 2 O 3 -Fe 2 O 3 -H 2 O.
2. The alkaline trend is found in dry to extremely dry zones of the earth, with a net annual movement of water upwards in the weathering profile. Aseasonal distribution of rainfall may cause a downward leaching at certain times of the year, but capillary uprise and evaporation of water are the distinctive features. Precipitation of groups I and II elements as carbonates, sulphates or halides is the result. With increasing aridity and evaporation, three main types of mineral concentrations are produced. First there is a near neutral calcitic type, with Ca 2+ and CO 3 -2 as notable ions. Second and more alkaline Mg 2+ , CO 3 -2 and SO 4 2- concentrated. Lastly a sodic, strongly alkaline type in which solutions rich in Na + , Ca 2+ , OH - , C1 - and SiO 2 may precipitate sodium carbonates, halite and chert. The calcitic type of pedogeneses is found in prairie soils, and the other two in aridic soil regimes.
3. The reducing trend occurs in hydromorphic environments of either predominantly inorganic (clay-rich lowlands) or organic (swamps, marshes) type. Low partial pressures of oxygen ensure a mobilization of Fe (and Mn) into the aqueous phase. The characteristic pedogenic process in low S 2- , low HCO 3 - environments, is gleying where Fe and Mn leave the profile in solution. Secondary minerals may form in high S 2- environments (e.g. pyrite) and in high HCO 3 - ones (e.g. siderite). Acid clays may be produced in a two stage process called ferrolysis. The first stage is a reduction to mobilize Fe 2+ which then replaces Ca 2+ and K + on exchange sites of clays. A later oxidation (perhaps caused by a lowered water table) then causes H + to replace Fe 2+ and acidify the clay. Fe (and Mn) may then precipitate as hydroxy-minerals, forming mottles, concretions or indurated layers (hardpan).
On release into the aqueous environment, behavior of the element and the mechanism controlling that behavior, can be succinctly expressed in a modification of the classic Goldschmidt-Mason diagram (Fig. 3).
In addition to chemical processes, two physical processes are also important in·reorganizing the geochemistry on and within a landscape. On the landscape erosional forces will tend to move the soil mantle downslope, while within the weathering profile physical entrainment of solids in the aqueous phase will move the clay fraction, plus absorbed elements down profile and downslope, to produce illuvial horizons.
APPLICATIONS OF THE PHASE RULE TO SOIL SYSTEMS
The basic problem in using the Phase Rule to set up an equilibrium model for weathering systems and soils in particular, is that such systems are of great compositional complexity. Consequently, there is a need to simplify as much as possible, while still retaining sufficient complexity to enable reasonable statements to be made about real systems. In particular, we need to know what components and what phases we should consider, and what range of environmental conditions are appropriate.
What are the important components? Over wide areas, the average composition of the earth's continental surface is andesitic. Since the crust is also essentially a close packed framework of oxygens it can be considered initially as being made up of oxide components such as SiO 2 , A1 2 O 3 , Fe 2 O 3 , CaO, MgO, Na 2 O and K 2 O as majors, and TiO 2 , MnO 2 and P 2 O 5 as minors. In addition important components from the atmosphere and hydrosphere are H 2 O, CO 2 and O 2 . The biosphere provides further complications in terms of organic components.
What are the important phases? The important phases that need to be modeled in a soil system are the ones that form there. These include the following: oxides and hydroxides (e.g. quartz, goethite, hematite, gibbsite and boehmite), 1:1 sheet silicates (e.g. kaolinite and halloysite), 2:3 sheet silicates (e.g. illite, smectite and vermiculate), 2:1:1 sheet silicates (e.g. hydroxy-interlayered vermiculite), framework silicates (e.g. zeolites and possibly feldspars), carbonates (e.g. calcite, siderite) and other minerals such as gypsum and halite. No single system contains all of these phases. Generally no more than two to four need be considered together, the specific soil environment under consideration dictating the choice.
What are the appropriate environ-mental conditions? The most generally useful master variables of the weathering environment are pe (or Eh) and pH. The spread of pe-pH conditions in the stability field of water, and found in nature is approximately as shown (Fig. 4). Ignoring a number of rather rare environments at the surface of the earth (e.g. acid sulphate soils, weathering vanadium deposits), the normally expected conditions cover a pe-pH field which shows three salients, each of which corresponds to one of the three lines of chemical evolution shown by soils in weathering.
The behavior of water dictates which trend is followed. The acid and alkaline trends are in the oxidizing (water-unsaturated) zone of weathering. The acid trend requires an excess of water with a net leaching or downward movement. The alkaline trend is found in dryer environments with a net loss of water by evapo-transpiration. A reduced trend is found in water-saturated conditions in a weathering profile.
The acid trend, the common one in weathering, is found in regions of humid climate on materials with a generally unimpeded drainage. Hydrolysis is the principal reaction, wherein acids from atmospheric and biospheric sources react within the solid earth as base. H + progressively displaces other cations, which are leached out of the system in solution. This acidification of the weathering materials pushes the soil towards the acid salient of figure 4 and soils such as podzols and ferralsols are produced. The result is an evolution of soils towards compositions made up chiefly of the four components SiO 2 -A1 2 O 3 -Fe 2 O 3 -H 2 O. This can be consi-dered the fundamental system in soil science since all four components dominate virtually all soils.
Figure 5 is a compatibility diagram of the fundamental system showing phase assem-blages stable at 25°C and 100 kPa. It is constructed stepwise from the constituent 1, 2 and 3 component systems. Projection from the H 2 O apex (Fig. 5b) displays those minerals that can coexist with water at equilibrium at the surface of the earth (assuming aH 2 O = 1). Since goethite is virtually a ubiquitous phase in soils in humid regions a projection from the Fe 2 O 3 apex yields a further simplification of the compatibility diagram (Fig. 5c). Finally the aqueous phase in the acid trend has a high mobility, and the chemical potentials of A1 and Si species in this phase may be considered as environmental variables, applied to the mineralogical system like temperature and pressure, from outside. In this case, the four component system under consideration can be depicted as in figure 3, with the additional information that goethite is also present in all fields. Earlier stages of the acid trend require a consideration of equilibria involving smectites and illites. In the simplest depiction a minimum of two more components must be added, and if these are chosen as MgO and K 2 O, figure 6 can be derived. The upper pH limit of the acid trend is found in Ca and Mg carbonate-bearing soils developed on limestones and dolomites. To some degree the carbonate-bearing system (e.g. figure 7) can be considered as separate from the alumino-silicate one, though the presence of CaO as a component will certainly affect the stability field of smectite in figure 6. One way of combining the aluminosilicate and carbonate subsystems is by means of the a-C-Fm diagram (Fig. 8).
The alkaline trend occurs in relatively arid climatic zones, where the net annual movement of water is upwards in the weathering zone. For part of the year, the seasonal distribution of rainfall may determine that there is enough water to effect a solution and downward leaching of soil components. However, the distinctive features of this pedogeneses, caused by capillary uprise and evaporation of soil water and evaporation and precipitation. The geochemical result is that a number of electrolytes, (e.g. Na + , Mg +2 , Ca +2 , HCO 3 - , CO 3 -2 , SO 4 -2 , C1 - , CO 3 - ) may reach high concentrations in the system, migrate in the profile, and produce precipitations of salts in certain horizons. An important feature of the solution phase will be that aH 2 O is significantly less than 1. The order in which mineral phases precipitate in such systems is determined by their solubility products. The early stages of this process can be followed in figure 9. Later stages may involve the precipitation of alkali carbonates and zeolites (figure 10). The ultimate path of mineralization will depend upon the composition of the soil solution at the start, with each precipitating salt acting as a chemical barrier separating pairs of evolutionary trends (Hardie and Eugster, 1970). In pedology, the process is called alkalinization or solonization, and is qualitatively similar to the formation of evaporties in, for example, the East African Rift. Alkalinized soils may later be modified by a change to a wetter climate, to produce assemblages (including zeolites) in figure 11.
The reduced trend in weathering is characterized by the presence of an excess of water. The weathering profile is completely or partially submerged, leading to a lowering of the partial pressure of oxygen in the system to the degree that anaerobic conditions may develop. Elements with multiple oxidation states will be reduced. In the case of Fe and Mn, the reduced forms are readily mobilized, a contrast to the behavior of these elements in oxidizing environments. Close to the water table, fluctuations in its level produce alternating redox conditions, with a resulting mobilization and changes.
The major visible changes are those associated with Fe. As the solvated ferrous ion, Fe may leave the system so that the soil loses the dark colors associated with the presence of iron-bearing solids, and acquires lighter colors tending towards grey, (a process known as gleying). Other features of the chemical environment may affect the behavior of Fe. If the environment contains sulphate ion and sulphate-reducing bacteria are present, pyrite may form. Where carbonate ions dominate, siderite may result. If sulphide and carbonate ions are scarce, but sufficient silica is present in solution, the authigenic solicate glauconite may appear. Figure 12 shows one of many possibilities. Brookins (1988) is a compendious reference for others.
PROBLEMS WITH THE APPLICATION OF THE PHASE RULE TO SOILS
The phase diagrams used by soil scientists are generally based on calculation from the fundamental thermodynamic data, rather than an experimentation as is the case in high PT geochemistry. The basic recipes for low PT calculations are clearly laid out by Lindsay (1979). The necessary data, tabulated for 25°C and 100 kPa, is continually being revised and should always be critically examined for internal consistency (Helgeson et al., 1978; Berman, 1988). It should be noted that tabulated data is almost invariably for simple, stoichiometric compositions. Isomorphous replacements (of Fe for A1 and vice versa, for example) may change stability fields significantly (Fig. 13.). Furthermore, 25°C and 100 kPa may not always be the best choice of conditions for pedogenic models. Other temperatures may be more appropriate, especially where diagenetic effects need to be considered, for example in paleosols (see fig. 14).
Construction of a phase diagram to illuminate a problem will lead to difficulties if the choice of components and phases to be considered is not a judicious one. The diagram itself is only valid for the phases considered, and the most important difference between phases in theoretical diagrams and phases in nature is the fact that the latter exhibit various structural states, crystallinities, and isomorphous substitutions (solid solution). In addition, metastable equilibria need to be considered as well as stable ones.
The most important single component in the soil system is H 2 O. Its activity is usually taken to be 1. However, there exists the possibility of aH 2 O being less than 1, for example, in saline environments. The effect of lowering aH 2 O will be to cause dehydration reactions to take place at lower temperatures than they would otherwise do (Fig. 15). In other words the result is to diminish the field of stability of the hydrated phase in the presence of an aqueous solution.
Mixed layer clay minerals present a further problem. Should they be considered single or multiple phases? Both points of view have been defended, though the most recent work based on 39 Si NMR studies suggest that mixed layer illite/smectite structures act thermodynamically as two phases (Altaner et al., 1988).
In soils, surface reactions are the rule, so that it may seem unwise to use the unmodified Gibbs' Phase Rule, which specifically ignores surface phenomena. It is possible that surface energy contributions in soil mineralogical reactions are of such a magnitude to change the product of reaction. Many important reactions involving common phases such as gibbsite, goethite or kaolinite, have DGr values close to zero, so that the surface contribution could be decisive. In figure 16, for example, kaolinite is the stable phase between pH 4 SiO 4 3 to 4, yet surface effects may result in metastable phases forming. Growth of such phases however, ultimately nullifies the surface effect (at, say, dimensions great than 10 -7 _m), and the metastable phase or phases may be replaced by more stable ones (the Ostwald Step Rule).
Altaner, S.P., Weiss, C.A. and Kirkpatrick, R.J. (1988). Evidence from 29 Si NMR for the structure of mixed layer illite/smectite clay minerals. Nature 331 699-702.
Berman, R.G. (1988). Internally consistent thermodynamic data for minerals in the system Na 2 O-K 2 O-CaO-MgO-FeO-Fe 2 O 3 -A1 2 O 3 -SiO 2 -TiO 2 -H 2 O-CO 2 . J. Petrology 29 445-522.
Bowers, T.S., Jackson, K.J., and Helgeson, H.C. (1984). Equilibrium Activity dia-grams. Springer-Verlag, Berlin, 397 p.
Brookins, D.G. (1988). Eh-pH diagrams for Geochemistry. Springer, Berlin, 176 p. Chesworth, W. (1992). Weathering systems: p. 19-40, in 'Weathering, Soils and Paleosols ed. by Martini, I.P. and Chesworth, W. Elsevier, N.Y. 619 p.
Chesworth, W. and Dejou, J. (1980). Are considerations of mineralogical equili-brium relevant to pedology? Evidence from a weathered granite in central France. Soil Science 130 290-292.
Connolly, J.A.D. (1990). Multivariable phase diagram: an algorithm based on generalized thermodynamics. Am. J. Sci. 290 666-718.
Defay, R., Prigogine, I., and Bellemans, A. (1966). Surface Tension and Adsorption. Longmans, London, 432 p.
Donnon, F.G. and Haas, A. (1936). A Com-mentary on the Scientific Writings of J. Willard Gibbs: V.I. Thermodynamics. Yale Univ. Press, New Haven, 742 p.
Eberl, D.D., Srodon, J., Kralik, M., Taylor, B.E. and Peterman, Z.E. (1990). Ostwald ripening of clays and metamorphic minerals. Science 248 474-477.
Eugster, H.P. and Hardie, L.A. (1970). Saline lakes: in A. Lerman (ed.) Lakes: Chemistry, Geology, Physics. Springer, N.Y., 363 p.
Garrels, R.M. and Christ, C.L. (1965). Solutions, Minerals and Equilibria. Harper and Row, N.Y., 450 p.
Gibbs, J.W. (1961). Collected Papers, V.I. Thermodynamics. Dover Books, N.Y. Korzhinskii, D.S. (1959). Physicochemical Basis on the Paragenesis of Minerals. Consultant Bureau, N.Y., 142 p.
Lindsay, W.L. (1979). Chemical Equilibria in Soils. Wiley and Sons, N.Y., 449 p.
Morel, F.M.M. (1983). Principles of Aquatic Chemistry. Wiley and Sons, N.Y., 446 p. Pankow, J.F. (1999). Aquatic Chemical Concepts. Lewis Publishers, Chelsea, MI. 673 p.
Prigogine, I., and Defay, R. (1954). Chemical Thermodynamics. Longmans, London, 522 p.
Ricci, J.E. (1951). The Phase Rule and Hete-rogeneous Equilibrium. Van Nostrand, N.Y., 505 p.
Rosenberg, P.E., Kittrick, J.A. and Aja, S.U. 1990. Mixed layer illite/smectite: a multi-phase model. Am. Miner. 75 1182-1185.
Schulman, D. and Chesworth, W. (1985). Calcium carbonate solubility in the c horizon of a southern Ontario, Canada, luvisol. Chemical Geol., 51 173-181.
Seeger, R.J. (1974). J. Willard Gibbs. Pergamon, Oxford, 290 p.
Spiers, G.A. Pawluk, S., and Dudas, M.J. (1984). Authigenic mineral formation by solonization. Can. J. Soil Sci., 64 515-532.
Sposito, G. (1989). The Chemistry of Soils. O.U.P., N.Y., 277 p.
Trolard, F. and Tardy, Y. (1989). A model of Fe 3+ -kaolinite, A1 3+ -goethite, A1 3+ -hematite equilibria in laterites. Clay Minerals 24 1-21.
Velde, B. (1985). Clay Minerals: a Physico-Chemical Explanation of their Occurren-ce. Elsevier, N.Y., 427 p. Zen, E-an. (1959). Clay mineral-carbonate relations in sedimentary rocks. Amer. J. Sci. 257 29-43.
Zen, E-an. (1963). Components, phases, and criteria of chemical equilibrium in rocks. Amer. J. Sci. 261 929-942.